The world of mathematics is filled with intriguing identities and trigonometric functions that challenge even the most seasoned scholars. One such expression that often perplexes students and enthusiasts alike is cos4pi 3. Understanding this function not only enhances your mathematical toolkit but also connects you to a broader understanding of trigonometry and its applications in real-life scenarios. In this article, we will dive deep into cos4pi 3, breaking down its components and exploring how to compute it effectively.
Trigonometric functions like cosine play a vital role in various fields, from physics and engineering to computer graphics and architecture. The expression cos4pi 3 is a specific instance of the cosine function, showcasing the periodic nature of trigonometric relationships. By grasping the intricacies of this expression, you will gain insights into how angles and their corresponding cosine values interact within the unit circle, which is foundational for any mathematical journey.
As we explore cos4pi 3, we will answer common questions, provide illustrative examples, and discuss its relevance in both theoretical and practical applications. Whether you're a student preparing for an exam or a professional brushing up on your math skills, understanding cos4pi 3 can enhance your comprehension of trigonometric concepts.
What is the Value of cos4pi 3?
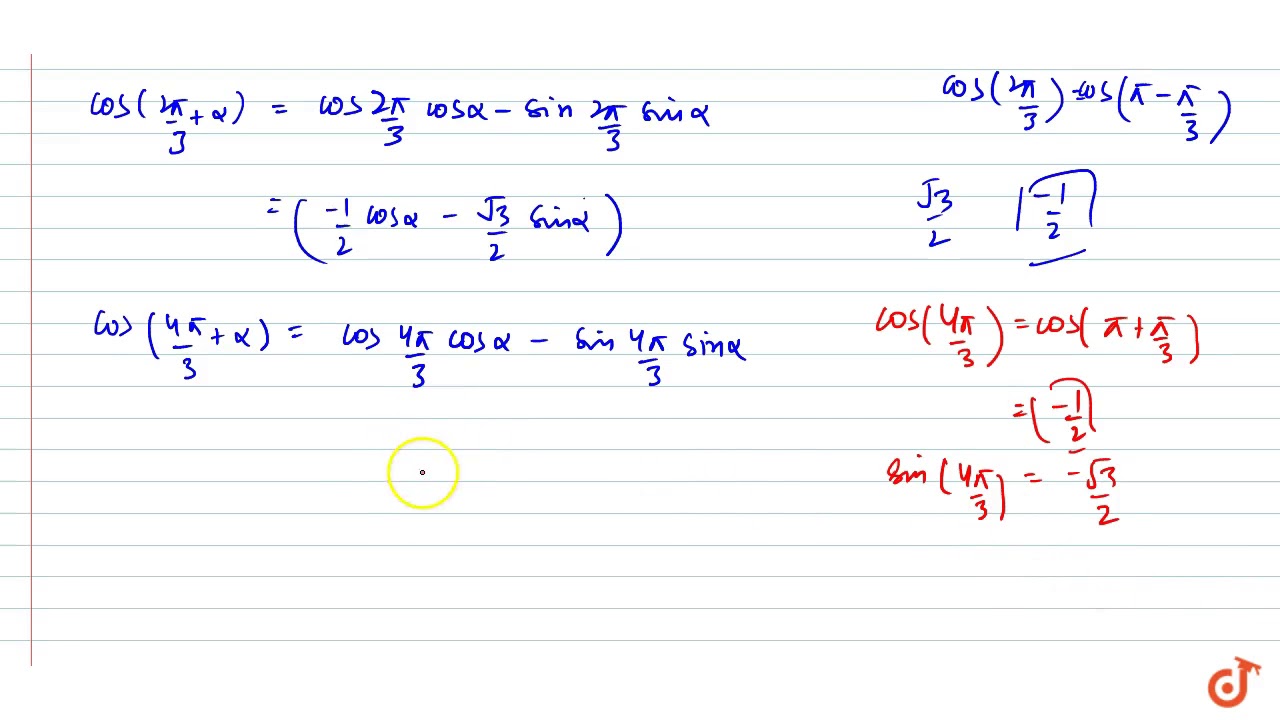
To compute cos4pi 3, we first need to understand the angle involved. The angle 4pi/3 radians can be converted to degrees for easier visualization. Since there are 180 degrees in pi radians, we can calculate:
- 4pi/3 radians = (4 * 180) / 3 = 240 degrees
Now, cos4pi 3 is equivalent to cos(240 degrees). From the unit circle, we know that 240 degrees lies in the third quadrant, where the cosine value is negative. Specifically, the reference angle for 240 degrees is 60 degrees, leading us to the cosine value:
- cos(240 degrees) = -cos(60 degrees) = -1/2
Thus, the final result for cos4pi 3 is:
- cos4pi 3 = -1/2
How is cos4pi 3 Related to the Unit Circle?
The unit circle is a fundamental concept in trigonometry that provides a graphical representation of angles and their corresponding sine and cosine values. The angle 4pi/3, or 240 degrees, corresponds to a specific point on the unit circle. By visualizing this angle, we can see that it extends 240 degrees counterclockwise from the positive x-axis.
In the unit circle:
- The x-coordinate represents the cosine value.
- The y-coordinate represents the sine value.
For the angle 240 degrees, the coordinates of the point on the unit circle are:
- (-1/2, -√3/2)
This reinforces our earlier computation that cos4pi 3 = -1/2.
Why is Understanding cos4pi 3 Important?
Understanding cos4pi 3 is crucial for several reasons:
- It enhances your grasp of periodic functions, especially cosine.
- It prepares you for more complex trigonometric equations and identities.
- It has applications in physics, engineering, and computer science.
What Are Some Practical Applications of cos4pi 3?
The value of cos4pi 3 may seem abstract, but it has practical significance in various fields. Here are some applications:
- Physics: In wave motion and oscillations, the cosine function is often used to describe displacement over time.
- Engineering: Cosine values are essential in calculating forces and moments in structures.
- Computer Graphics: Cosine functions are used in rendering images and animations, particularly in calculating lighting and shading.
How Can We Graph cos4pi 3?
Graphing cos4pi 3 involves plotting the cosine function over a range of angles. The cosine function is periodic with a period of 2pi, meaning it repeats every 360 degrees. To graph it:
- Plot points for key angles, such as 0, pi/2, pi, 3pi/2, and 2pi.
- Mark the corresponding cosine values for these angles.
- Connect the points to form a smooth wave, showcasing the oscillating nature of the cosine function.
Can We Solve for Other Trigonometric Functions Using cos4pi 3?
Yes! Knowing the value of cos4pi 3 allows us to derive other trigonometric functions, such as sine and tangent. Since we already established:
- cos(240 degrees) = -1/2
- sin(240 degrees) = -√3/2
We can compute the tangent value using the formula:
- tan(θ) = sin(θ) / cos(θ)
For 240 degrees, this results in:
- tan(240 degrees) = (-√3/2) / (-1/2) = √3
What Are the Key Takeaways About cos4pi 3?
In summary, understanding cos4pi 3 is essential for grasping the broader concepts of trigonometry. Here are the key takeaways:
- cos4pi 3 = -1/2, a value derived from the cosine function.
- The angle corresponds to 240 degrees in the unit circle, where cosine values are negative.
- Knowledge of this expression enhances your mathematical understanding and has practical applications across various fields.
By exploring the intricacies of cos4pi 3, you gain not only a valuable mathematical skill but also a deeper appreciation for the beauty and complexity of trigonometry. Whether in an academic setting or real-world application, this knowledge will serve you well in your mathematical endeavors.
You Might Also Like
Unveiling The World Of The Extremely Thin: A Deep DiveEnchanting Elegance: The Adult Glinda Wizard Of Oz Costume
Unveiling The Meaning Of Spirit Animal: A Journey Into Inner Wisdom
Flirting With Physics: The Art Of Science Chat Up Lines
Embracing Life: The Journey Of A Mid 40's Japanese Woman
Article Recommendations
- Stunning Lizzie Snapgod Photos Videos
- Exploring The World Of Masa49 Video A Comprehensive Guide
- The Enigmatic World Of The Plumpy Mage A Deep Dive Into Mystical Realms