Understanding The Numerical Aperture Of Optical Fiber: A Comprehensive Guide

The numerical aperture of optical fiber is a crucial concept that plays a significant role in the performance and efficiency of fiber optic communication systems. As technology advances and the demand for high-speed data transmission increases, understanding the principles behind optical fibers has become more important than ever. This article delves into the intricacies of the numerical aperture, exploring its definition, significance, calculation, and impact on fiber optic systems.

In essence, the numerical aperture of optical fiber is a dimensionless number that quantifies the light-gathering ability of the fiber. It serves as an indicator of how much light the fiber can accept and transmit effectively, influencing the overall performance of the optical system. A higher numerical aperture typically allows for more light to enter the fiber, resulting in better signal quality and longer transmission distances. Thus, understanding this parameter is vital for designing efficient fiber optic networks.

Furthermore, the numerical aperture is not just a theoretical concept; it has practical implications for various applications, ranging from telecommunications to medical imaging and industrial sensing. By grasping the fundamentals of the numerical aperture of optical fiber, engineers and technicians can make informed decisions about the types of fibers to use, the configurations of optical systems, and the expected performance outcomes in real-world scenarios.

What is the Numerical Aperture of Optical Fiber?

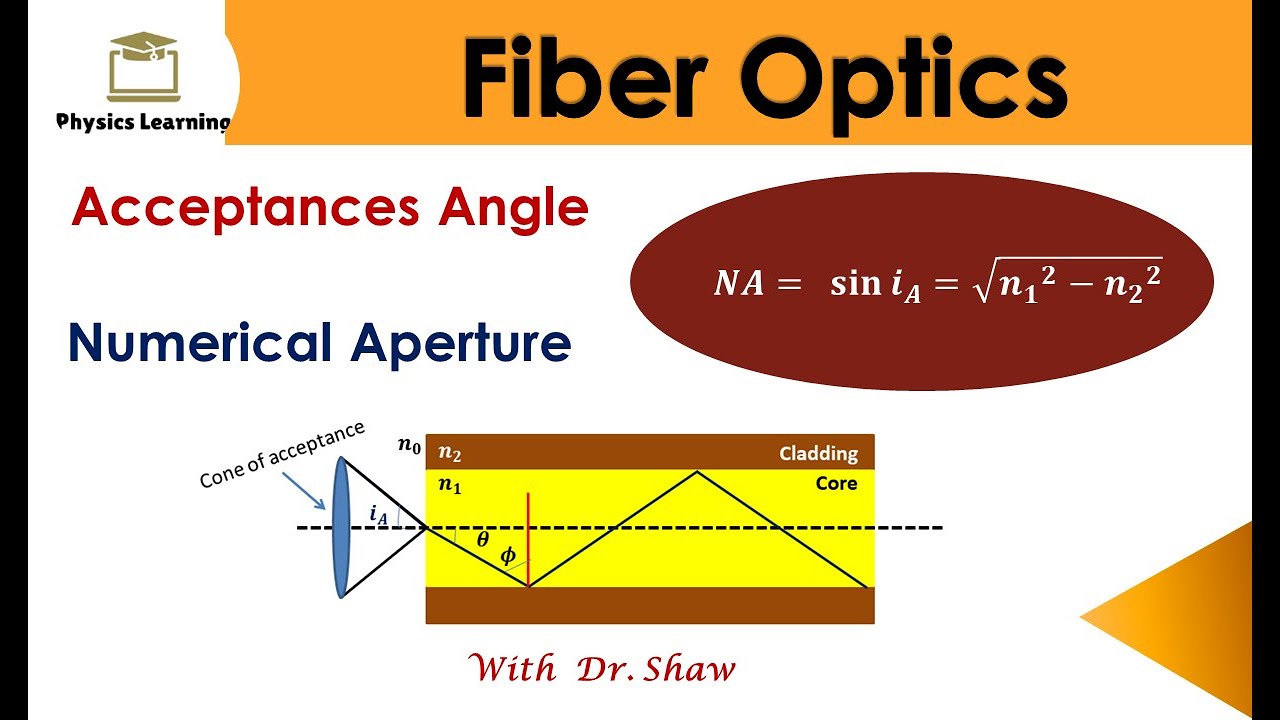
The numerical aperture (NA) of optical fiber is defined as the sine of the maximum angle at which light can enter the fiber and still be guided within the core. Mathematically, it is expressed as:

NA = n * sin(θ)

Where:

• n = refractive index of the core material
• θ = maximum acceptance angle

The NA determines how much light the optical fiber can collect and transmit, which is essential for minimizing loss and maximizing signal strength.

How is the Numerical Aperture Calculated?

To calculate the numerical aperture of an optical fiber, you need to know the refractive indices of the core and the cladding. The formula for NA in terms of the refractive indices is:

NA = √(n_core² - n_cladding²)

Where:

• n_core = refractive index of the core
• n_cladding = refractive index of the cladding

This calculation shows how the difference in refractive index between the core and cladding affects the fiber's ability to guide light.

Why is the Numerical Aperture Important in Optical Fiber?

The numerical aperture of optical fiber is essential for several reasons:

• Light Gathering Capability: A higher NA allows for more light to be captured and transmitted, which is vital for high-performance applications.
• Transmission Distance: Fibers with a higher numerical aperture can transmit signals over longer distances with less attenuation.
• Modal Dispersion: The NA affects the number of modes that can propagate through the fiber, influencing signal integrity and quality.
• Application Suitability: Different applications, such as telecommunications and medical devices, may require specific NA values for optimal performance.

What are the Types of Numerical Aperture in Optical Fibers?

There are generally two types of numerical aperture: step-index and graded-index. Each type has unique characteristics and applications.

Step-Index Fibers

Step-index fibers have a uniform core refractive index and a sudden change (step) to a lower refractive index in the cladding. They typically have a lower numerical aperture compared to graded-index fibers but are simpler to manufacture and work well for short-distance applications.

Graded-Index Fibers

Graded-index fibers feature a gradual change in refractive index from the core to the cladding. This design reduces modal dispersion, allowing for higher data rates over longer distances. These fibers often have a higher numerical aperture, making them suitable for high-performance applications.

How Does the Numerical Aperture Affect Signal Quality?

The numerical aperture plays a critical role in determining the signal quality transmitted through optical fibers. A higher numerical aperture generally means better light-gathering capabilities, which can lead to:

• Improved Signal-to-Noise Ratio: More light entering the fiber results in a stronger signal, making it less susceptible to noise.
• Reduced Bit Error Rate: Better signal quality reduces the likelihood of errors in data transmission.
• Enhanced Bandwidth: A higher NA can support greater bandwidth, facilitating faster data rates.

What are the Applications of Optical Fibers with Different Numerical Apertures?

Different applications require different numerical aperture values to optimize performance:

Telecommunications

In telecommunications, fibers with a higher numerical aperture are commonly used for long-distance data transmission, ensuring minimal signal loss and high bandwidth.

Medical Imaging

Medical imaging applications often utilize graded-index fibers due to their superior light transmission properties, optimizing image quality and detail.

Industrial Sensing

In industrial settings, optical fibers with varying numerical apertures are used for sensors, allowing for precise measurements and monitoring of environmental conditions.

Conclusion: The Importance of Understanding Numerical Aperture of Optical Fiber

In conclusion, the numerical aperture of optical fiber is a fundamental parameter that significantly influences the performance of fiber optic systems. By understanding its definition, calculation, and implications, engineers and technicians can design more efficient and effective optical networks. As technology continues to evolve, the importance of the numerical aperture in various applications will only grow, making it a vital topic for anyone involved in the field of optics.